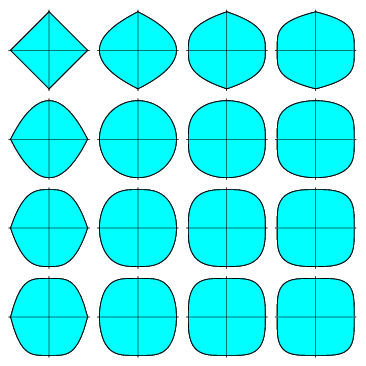
What is a superellipse
A superellipse, also known as a Lamé curve after Gabriel Lamé, is a closed curve resembling the ellipse, retaining the geometric features of semi-major axis and semi-minor axis, and symmetry about them, but a different overall shape.
In the Cartesian coordinate system, the set of all points (x, y) on the curve satisfy the equation
where n, a and b are positive numbers, and the vertical bars | | around a number indicate the absolute value of the number.

a = 1 and b = 0.75
There are many number of specific cases of superellipse like given in the image down below:
These can be achieved by varying the value of n in the equation. So now we try to implement this in python and to do that we are require some libraries.
Modules Required:
- matplotlib: To plot the curve of the equation. Its an 3rd party library in python and if you want to install it click here.
- math : Its an built in library of python which have almost all the mathematical tools.
# Python program to implement # Superellipse # importing the required libraries import matplotlib.pyplot as plt from math import sin, cos, pi def sgn(x): return ((x>0)-(x<0))*1 # parameter for marking the shape a, b, n = 200, 200, 2.5na = 2 / n # defining the accuracy step = 100 piece =(pi * 2)/step xp =[];yp =[] t = 0for t1 in range(step + 1): # because sin ^ n(x) is mathematically the same as (sin(x))^n... x =(abs((cos(t)))**na)*a * sgn(cos(t)) y =(abs((sin(t)))**na)*b * sgn(sin(t)) xp.append(x);yp.append(y) t+= piece plt.plot(xp, yp) # plotting all point from array xp, yp plt.title("Superellipse with parameter "+str(n)) plt.show() |
Output:

when n = 2.5
Now let’s see what happens when we changes the value of n to 0.5
# Python program to implement # Superellipse # importing the required libraries import matplotlib.pyplot as plt from math import sin, cos, pi def sgn(x): return ((x>0)-(x<0))*1 # parameter for marking the shape a, b, n = 200, 200, 0.5na = 2 / n # defining the accuracy step = 100 piece =(pi * 2)/step xp =[];yp =[] t = 0for t1 in range(step + 1): # because sin ^ n(x) is mathematically the same as (sin(x))^n... x =(abs((cos(t)))**na)*a * sgn(cos(t)) y =(abs((sin(t)))**na)*b * sgn(sin(t)) xp.append(x);yp.append(y) t+= piece plt.plot(xp, yp) # plotting all point from array xp, yp plt.title("Superellipse with parameter "+str(n)) plt.show() |
Output:
Source Code of the program in Java.
// Java program to implement // Superellipse import java.awt.*; import java.awt.geom.Path2D; import static java.lang.Math.pow; import java.util.Hashtable; import javax.swing.*; import javax.swing.event.*; public class SuperEllipse extends JPanel implements ChangeListener { private double exp = 2.5; public SuperEllipse() { setPreferredSize(new Dimension(650, 650)); setBackground(Color.white); setFont(new Font("Serif", Font.PLAIN, 18)); } void drawGrid(Graphics2D g) { g.setStroke(new BasicStroke(2)); g.setColor(new Color(0xEEEEEE)); int w = getWidth(); int h = getHeight(); int spacing = 25; for (int i = 0; i < w / spacing; i++) { g.drawLine(0, i * spacing, w, i * spacing); g.drawLine(i * spacing, 0, i * spacing, w); } g.drawLine(0, h - 1, w, h - 1); g.setColor(new Color(0xAAAAAA)); g.drawLine(0, w / 2, w, w / 2); g.drawLine(w / 2, 0, w / 2, w); } void drawLegend(Graphics2D g) { g.setColor(Color.black); g.setFont(getFont()); g.drawString("n = " + String.valueOf(exp), getWidth() - 150, 45); g.drawString("a = b = 200", getWidth() - 150, 75); } void drawEllipse(Graphics2D g) { final int a = 200; // a = b double[] points = new double[a + 1]; Path2D p = new Path2D.Double(); p.moveTo(a, 0); // calculate first quadrant for (int x = a; x >= 0; x--) { points[x] = pow(pow(a, exp) - pow(x, exp), 1 / exp); // solve for y p.lineTo(x, -points[x]); } // mirror to others for (int x = 0; x <= a; x++) p.lineTo(x, points[x]); for (int x = a; x >= 0; x--) p.lineTo(-x, points[x]); for (int x = 0; x <= a; x++) p.lineTo(-x, -points[x]); g.translate(getWidth() / 2, getHeight() / 2); g.setStroke(new BasicStroke(2)); g.setColor(new Color(0x25B0C4DE, true)); g.fill(p); g.setColor(new Color(0xB0C4DE)); // LightSteelBlue g.draw(p); } @Override public void paintComponent(Graphics gg) { super.paintComponent(gg); Graphics2D g = (Graphics2D)gg; g.setRenderingHint(RenderingHints.KEY_ANTIALIASING, RenderingHints.VALUE_ANTIALIAS_ON); g.setRenderingHint(RenderingHints.KEY_TEXT_ANTIALIASING, RenderingHints.VALUE_TEXT_ANTIALIAS_ON); drawGrid(g); drawLegend(g); drawEllipse(g); } @Override public void stateChanged(ChangeEvent e) { JSlider source = (JSlider)e.getSource(); exp = source.getValue() / 2.0; repaint(); } public static void main(String[] args) { SwingUtilities.invokeLater(() -> { JFrame f = new JFrame(); f.setDefaultCloseOperation(JFrame.EXIT_ON_CLOSE); f.setTitle("Super Ellipse"); f.setResizable(false); SuperEllipse panel = new SuperEllipse(); f.add(panel, BorderLayout.CENTER); JSlider exponent = new JSlider(JSlider.HORIZONTAL, 1, 9, 5); exponent.addChangeListener(panel); exponent.setMajorTickSpacing(1); exponent.setPaintLabels(true); exponent.setBackground(Color.white); exponent.setBorder(BorderFactory.createEmptyBorder(20, 20, 20, 20)); Hashtable<Integer, JLabel> labelTable = new Hashtable<>(); for (int i = 1; i < 10; i++) labelTable.put(i, new JLabel(String.valueOf(i * 0.5))); exponent.setLabelTable(labelTable); f.add(exponent, BorderLayout.SOUTH); f.pack(); f.setLocationRelativeTo(null); f.setVisible(true); }); } } |
Output:
Reference Links:
1. Wikipedia – Superellipse
2. WolframMathWorld – Superellipse
This article is contributed by Subhajit Saha. If you like neveropen and would like to contribute, you can also write an article using write.geeksforgeeks.org or mail your article to review-team@geeksforgeeks.org. See your article appearing on the neveropen main page and help other Geeks.
Please write comments if you find anything incorrect, or you want to share more information about the topic discussed above.
Ready to dive in? Explore our Free Demo Content and join our DSA course, trusted by over 100,000 neveropen!